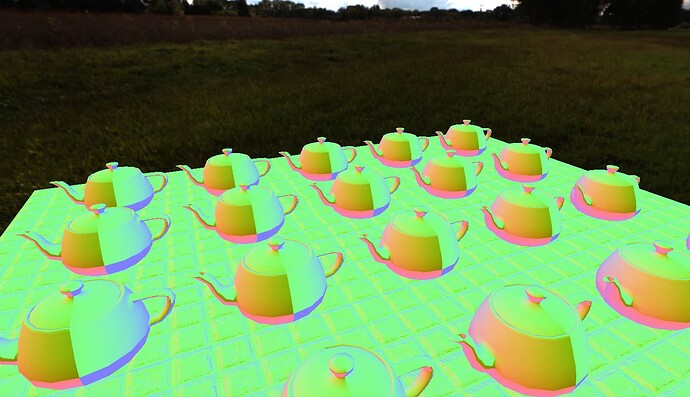
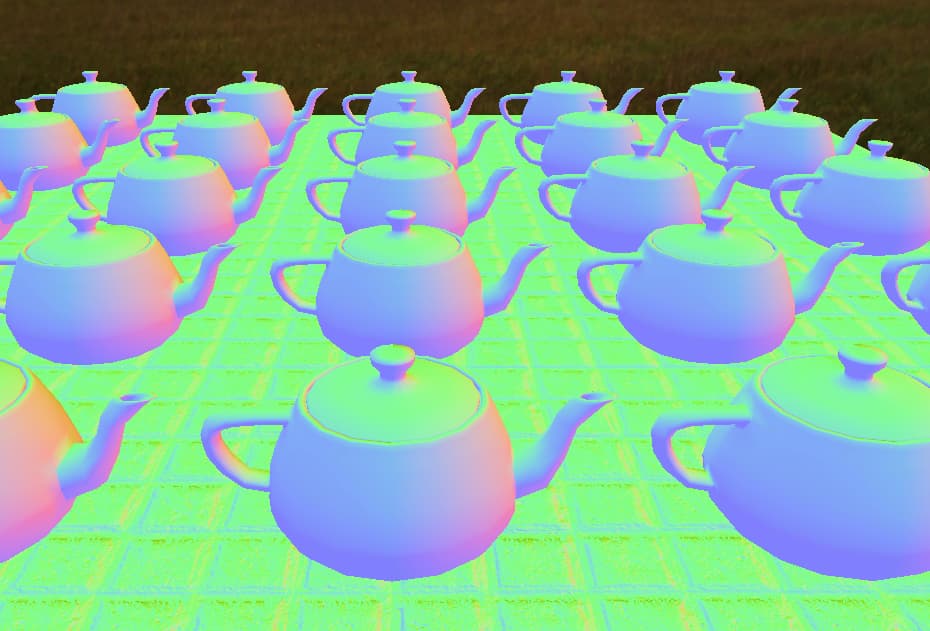
This are some GLSL functions to pack and unpack 3 component normals to 2 components.
They are based on A Survey of Efficient Representations for Independent Unit Vectors.
This is mostly useful for packing normals into a GBuffer. You should pack the normals into 2 16bit floating point channels. I also included some #if statements if you don’t have GLSL 4.00 available.
Usage is pretty straightforward:
// Packing the normal to 2 components
vec2 packed_normal = pack_normal_octahedron(my_normal);
// Unpacking the normal from 2 components
vec3 unpacked_normal = unpack_normal_octahedron(packed_normal);GLSL Functions:
/*
Normal packing as described in:
A Survey of Efficient Representations for Independent Unit Vectors
Source: http://jcgt.org/published/0003/02/01/paper.pdf
*/
// For each component of v, returns -1 if the component is < 0, else 1
vec2 sign_not_zero(vec2 v) {
#if 1
// Branch-Less version
return fma(step(vec2(0.0), v), vec2(2.0), vec2(-1.0));
#else
// Version with branches (for GLSL < 4.00)
return vec2(
v.x >= 0 ? 1.0 : -1.0,
v.y >= 0 ? 1.0 : -1.0
);
#endif
}
// Packs a 3-component normal to 2 channels using octahedron normals
vec2 pack_normal_octahedron(vec3 v) {
#if 0
// Version as proposed by the paper
// Project the sphere onto the octahedron, and then onto the xy plane
vec2 p = v.xy * (1.0 / (abs(v.x) + abs(v.y) + abs(v.z)));
// Reflect the folds of the lower hemisphere over the diagonals
return (v.z <= 0.0) ? ((1.0 - abs(p.yx)) * sign_not_zero(p)) : p;
#else
// Faster version using newer GLSL capatibilities
v.xy /= dot(abs(v), vec3(1));
#if 0
// Version with branches
if (v.z <= 0) v.xy = (1.0 - abs(v.yx)) * sign_not_zero(v.xy);
return v.xy;
#else
// Branch-Less version
return mix(v.xy, (1.0 - abs(v.yx)) * sign_not_zero(v.xy), step(v.z, 0.0));
#endif
#endif
}
// Unpacking from octahedron normals, input is the output from pack_normal_octahedron
vec3 unpack_normal_octahedron(vec2 packed_nrm) {
#if 1
// Version using newer GLSL capatibilities
vec3 v = vec3(packed_nrm.xy, 1.0 - abs(packed_nrm.x) - abs(packed_nrm.y));
#if 1
// Version with branches, seems to take less cycles than the
// branch-less version
if (v.z < 0) v.xy = (1.0 - abs(v.yx)) * sign_not_zero(v.xy);
#else
// Branch-Less version
v.xy = mix(v.xy, (1.0 - abs(v.yx)) * sign_not_zero(v.xy), step(v.z, 0));
#endif
return normalize(v);
#else
// Version as proposed in the paper.
vec3 v = vec3(packed_nrm, 1.0 - dot(vec2(1), abs(packed_nrm)));
if (v.z < 0)
v.xy = (vec2(1) - abs(v.yx)) * sign_not_zero(v.xy);
return normalize(v);
#endif
}